Protection from Radiations
THE RELATIONSHIP BETWEEN THE DISTANCE FROM SOURCES OF RADIATION AND SAFETY - THE "LAW OF THE INVERSE SQUARE" IN EMF / RF RADIATIONS
The inverse square law states that the magnitude of one's exposure drops dramatically, proportionally to the square of one's distance from the source.
Conversely, the magnitude of one's exposure also increases dramatically with proximity.
The following tables tell you the approximate distances required to reach the Salzburg Precautionary Limit of 2001 and 2002. The purpose of the calculations below is to demonstrate that the inverse square law (defined at right) is not as helpful as you may think, when the level at which health effects occur is far below the transmit power of the devices (and international standards).
After performing the calculations, one realizes that having a cordless phone station using DECT non-stop radiation, or a Wi-Fi router in the same room provides similar (or greater) power densities to living near a cell tower. Sleeping with an alarm clock within one foot of your head is like having one's personal electricity tower. There are studies showing that both cell towers (e.g., see studies by Robert Santini) and cordless phones (e.g., see studies by Leonnart Hardell) can be a potential health risk. Effects have been reported at very low levels.
Below, find the comparisons between:
- Wi-Fi devices, Cordless Phones, and Mobile phones and Smart Meters, Cell Towers, and Radio Towers
- Alarm Clock and Power Lines
Note that the measurements below assume radiation in direct line of sight. Wood and dry wall do very little to block wireless signals. Windows with low-E coating or low-E films, concrete, and brick, may provide higher levels of attenuation, and are not factored into the calculations below
Wi-Fi Router (microwave exposure) with transmit power (30mW)

Relashionship of Distance from Radiation Sources and Safety - the "LAW OF THE INVERSE SQUARE"
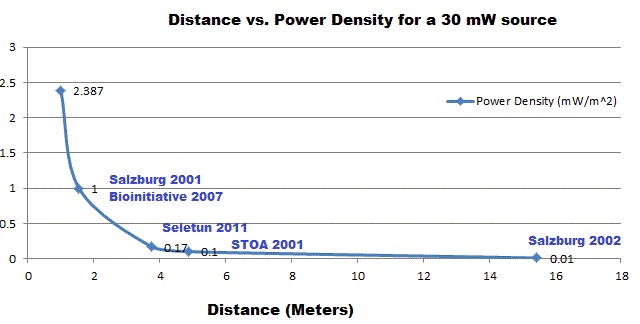
| Distance | Approx. Power Density** | Notes |
|---|---|---|
| 30cm | 26.5mW/m2 | Calculation: 30/4Π(0.3*0.3) =~26.5 |
| 1m | 2.387 mW/m2 | Calculation: 30/4Π =~2.387 |
| 1.55m | 1 mW/m2 | Salzburg Precautionary Limit (2001) BioInitiative Recommendation — (2007) |
| 3.7m | 170 microW/m2 | Seletun Scientific Panel (2011) |
| 5m | 100 microW/m2 | STOA (2001) |
| 15.5m | 10 microW/m2 | New Salzburg Precautionary Outdoor (2002) |
Method of Calculation: We can approximate the average power density using the equation P*G/4Πr2, where 4Πr2 is the surface area of a sphere and G is the antenna gain. For approximate purposes, the numbers calculated below assume an isotropic antenna with uniform intensity.
**Note that directional antennae are not factored in here, which could underestimate the power in certain directions and overestimate the power in other directions. For directional antennae, we must factor in gain to calculate the maximum required distance. The distances in this section may need to be additionally adjusted by multiplying the distances by the square root of the gain. This multiplication factor might vary anywhere from 1.3 to 7, depending upon the antenna's gain.
The following presentation by Graham Philips shows that a Wi-Fi enabled laptop at 50 cm can provide more radiation than a mobile phone mast (cell tower) at 100 meters (See slide 13).
Recommended Distances for Wireless Devices
The following table shows the minimal distances required to satisfy the Salzburg 2001 Limit and 2002 Outdoor Limit based upon the assumption of an isotropic antenna. According to the Table of Effects mentioned above, some effects are still seen at the Salzburg(2001) limit. Hence, the Salzburg (2002) limit is preferrable, or somewhere in between. To calculate these numbers yourself given the transmit power of a wireless product, refer to the Formulas page.
**Note: Since the antenna may be directional rather than isotropic, the distances below may be higher depending upon the direction and the antenna gain. In some directions, the distances required could be anywhere from 1.3 to 7 times higher depending upon the antenna's gain.
These are only general guidelines. Different wireless routers will vary in strength. Some wireless routers allow you to reduce the transmitting power, and even schedule the hours during which Wi-Fi will be turned on.
| Distance | Approx. Power Density** | Notes |
|---|---|---|
| 30cm | 26.5mW/m2 | Calculation: 30/4Π(0.3*0.3) =~26.5 |
| 1m | 2.387 mW/m2 | Calculation: 30/4Π =~2.387 |
| 1.55m | 1 mW/m2 | Salzburg Precautionary Limit (2001) BioInitiative Recommendation — (2007) |
| 3.7m | 170 microW/m2 | Seletun Scientific Panel (2011) |
| 5m | 100 microW/m2 | STOA (2001) |
| 15.5m | 10 microW/m2 | New Salzburg Precautionary Outdoor (2002) |
| Device | Duration Transmit Power |
Salzburg (2001)** |
Salzburg (2002)** |
Comments |
|---|---|---|---|---|
 Wi-Fi Routers (Lower Power) Wi-Fi Routers (Exterior AP) Wi-Fi Routers (High Power) |
Continual from router or access point, even when not in use 30 mW (~15dBm) 63 mW (~18dBm) 500 mW (~27dBm) |
1.55m 2.24m 6.3m |
15.5m 22.4m 63m |
Note that Wi-Fi enabled computers may be similar in strength to 15 dBm. If possible, choose a Wi-Fi router with configurable strength and scheduling ability. For example, one particular model provides 10 levels of power, allowing one to drastically reduce the strength. Wi-Fi routers and Digital Cordless Systems are allowed up to a transmit power as high as 1W (requiring 9m/90m recommendations for Salzburg (2001) and (2002) respectively. |
| Cordless Phone System (Earlier Analog) | Standby from base station, when not in use ~1mW |
0.3m | 3m | Note that very early cordless phones were by comparison only ~1mW, but now the FCC allows a higher transmitter power of 1W. See reference. |
| Cordless Phone System (Modern Digital) |
Continual from base station, even when not in use Peak: ~250mW Mean: ~10mW |
4.5m | 45m | While the average output power is ~10mW, it is in the form of 100 bursts per second of 250 mW power, each for ~0.4 ms, according to HPA on Cordless Phones. Hence, its peak output power is comparable to a mobile phone. The pulsing on/off signals are believed to be dangerous. |
| Mobile Phone |
Settings determine duration of exposure ~125mW (power class 4) ~250 mW (power class 3) 600mW Adaptive power |
3.2m 4.5m 6.9m |
32m 45m 69m |
To reduce the duration of exposure, turn off data services like >"Mobile Data" and "Wi-Fi" except when needed, turn off Background Data, or increase auto-sync intervals, and avoid use where signal reception is weak. Although speaker mode or a wired headset at 1 meter's distance greatly reduces the power density, it may not be enough to achieve the precautionary limit. By adaptive power control, a cell phone may adjust to a lower power level based on proximity to the nearest base station. |
See Wikipedia site on relative strengths of wireless transmissions.
Smart Meter (at 1 W, or 2.5 W after factoring in gain)
| Distance | Approximate power density assuming 4 dBi gain |
Notes |
|---|---|---|
| 1m | 199 mW/m2 | 1000mW*10^0.4/(4Π(1m)2)=199 mW/m2 |
| 3m | 22 mW/m2 | |
| 14m | 1 mW/m2 | <== Salzburg Precautionary Limit (2001) |
| 44m | 100 μW/m2 | <== EU STOA 2001 |
| 140m | 10 μW/m2 | <== New Salzburg Precautionary Outdoor (2002) |
Method of Calculation: The power density is estimated using the equation P*G/4Πr2, where G is the gain from the directional antenna (4 dBi translates to 10^(4/10)=2.5) and 4Πr2 is the surface area of a sphere.
See an actual smart meter measurement from Stop Smart Meters!. Note that the meter used in this video uses μW/cm2. To convert from μW/cm2to mW/m2, multiply by 10. Thus, 8 to 40 μW/cm2 converts to 80 to 400 mW/m2. For more information on smart meters, see What is a Smart Meter?.
2b. Cell Tower/Mobile Phone Mast (10 W)
| Distance | Approximate power density assuming 17 dB gain |
Notes |
|---|---|---|
| 100m | 4 mW/m2 | 10000mW/(4Π(100m)2)*10^(17/10)=0.08*50=4 mW/m2 |
| 200m | 1 mW/m2 | <== Salzburg Precautionary Limit (2001) |
| 630m | 100 μW/m2 | <== EU STOA 2001 |
| 2 km | 10 μW/m2 | <== New Salzburg Precautionary Outdoor (2002) |
Method of Calculation: The power density can be estimated using the equation P*G/4Πr2, where G is the gain from the directional antenna and 4Πr2 is the surface area of a sphere. In practice, the cell tower radiation is not usually isotropic but is directional with main lobe and side lobes. Note that a directional antenna focuses the power in certain directions, resulting in higher required distances. If we assume a directional antenna with gain of 17 dB = 50, then the distances required in certain directions could require a multiplication factor of sqrt(50)=7. For more information, see Report on Cell Tower Radiation submitted to Secretary, DOT, Delhi, Prepared by Prof. Girish Kumar, IIT.
Recommended Distances for Wireless Towers
Note: Many surveys find cancer or other health effects within 300-400 meters.
| Transmitter | Transmit Power assume 17dB gain |
Salzburg (2001)* |
Salzburg (2002)* |
|---|---|---|---|
| Very Weak Transmitter | 1 W | 63m | 630m |
| Weak Cell Tower | 10 W | 200m | 2 km |
| Strong Cell Tower | 50 W | 446m | 4.46 km |
| Stronger Cell Tower | 100 W | 630m | 6.3 km |
- Note: If there are more transmitters, the power output can be higher. For example, the HPA UK website suggests that ten 10W transmitters could result in a power output from 10 to 100 W.
- Note*: The calculations above assume a 17 dB gain (a factor of 10^1.7=50) as suggested by Professor Kumar. To accommodate this, the distance is multipled by a factor of approximately sqrt(50)=7, as compared to an isotropic antenna model.
3. Radio Tower
Note: Vatican radio tower study found cancer risk within a 5.5 mile radius = 8.85 km. The radio tower is said to include a 500 kW transmitter for the Far East and Latin America.
| Radio Tower | Transmit Power | Salzburg (2001) |
Salzburg (2002) |
|---|---|---|---|
| Radio Tower | 6 kW | 690m | 6900m |
| Radio Tower | 20 kW | 1.26 km | 12.6 km |
| Strong Radio Tower | 100 kW | 2.82 km | 28.2 km |
| Stronger Radio Tower | 500 kW | 6.3 km | 63 km |
| Very Strong Radio Tower | 1000 kW | 8.9 km | 89.2 km |
Method of Calculation: We can approximate the power density using the equation P*G/4Πr2, where 4Πr2 is the surface area of a sphere and G is the antenna gain. Below we will assume an omnidirectional isotropic antenna with G=1.
4. Alarm Clock (Power Line Exposure)
Note that power line (AC) magnetic fields drop much more quickly down to levels thought to be safe.
| Distance | Approximate magnetic field | Notes |
|---|---|---|
| 3 in. | 29.2 mG | Measured by Bell 4180 gaussmeter |
| 6 in. | 7.5 mG | |
| 9 in. | 3.1 mG | Childhood Cancer epidemiology studies |
| 12 in. | 1.6 mG | Infertility study. Ideally < 1mG |
| 16 in. | 0.8 mG | Better |
| 19 in. | 0.5 mG | Even better |
- Comment: The reduction typically occurs rapidly with a meter of distance. Meanwhile, the wireless power density reduction of 100,000+ times needed to satisfy the recommended safety limits, requires many meters.
5. Power Lines
| Power Line Source | Approximate Distance to reach 2mG |
|---|---|
| Alarm clock(above) | Between 9 and 12 inches |
| 11 kV lines | ~25m |
| 66 kV lines | ~50m |
| Strongest 400 kV lines | ~150m |
These approximations are taken from "Buying an "EMF Safe" Property - 2. Powerlines and pylons" from Powerwatch UK and the PowerWatch Handbook, p.43. However, it is stressed that one should measure the magnetic field to be sure. Even in the absence of power lines, there are other factors that can also influence the power line magnetic field.
Furthermore, note that ideally, the target level should be < 1 mG, with an even stricter guideline of < 0.2 mG for sleeping quarters. See the Power line Fact Sheet and the SBM 2008 standard for power lines which recommend levels < 1 mG.
Inverse-square law Wikipedia Article
We shield Buildings / Spaces and we detect Electromagnetic Fields that put areas, settlements and buildings due to the existence of Antenna Parks in the area ...




